Between any two rationals there is an irrational.
 •
211 Decimal
•
211 Decimal
The decimal numeral system (base-ten positional numeral system, denary or decanary) is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic numeral system.
9705#9712
SIBLINGS
CHILDREN
9712

-


 •
21 Fundamental theorem of arithmetic
•
21 Fundamental theorem of arithmetic
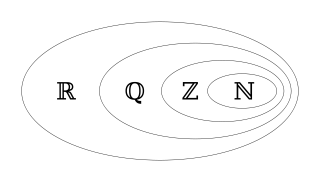 •
21 Rational number
•
21 Rational number
 •
211 Decimal
•
211 Decimal
 •
223
•
223